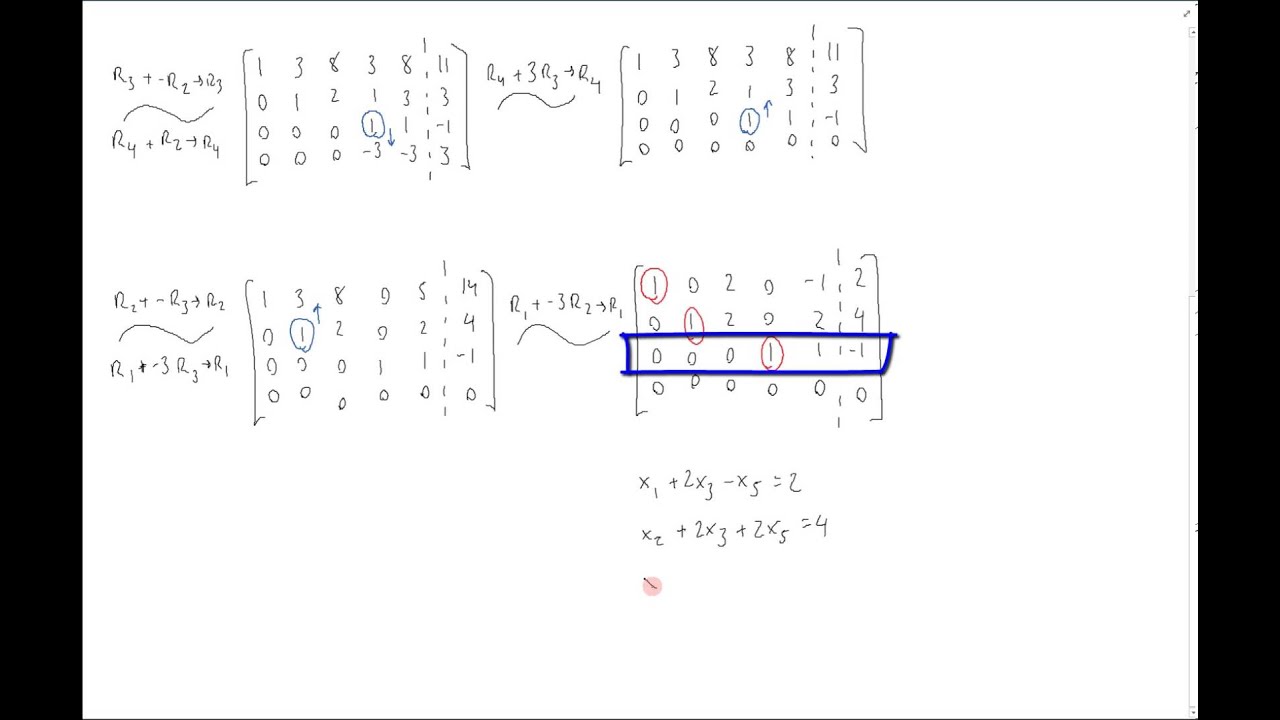
Parametric Vector Form Matrix

Parametric Vector Form Matrix - The parameteric form is much more explicit: Describe all solutions of $ax=0$ in parametric vector form, where $a$ is row equivalent to the given matrix. You can choose any value for the free variables. It gives a concrete recipe for producing all solutions. This is called a parametric equation or a parametric vector form of the solution. Suppose that the free variables in the homogeneous equation ax. A common parametric vector form uses the free variables. Parametric vector form (homogeneous case) let a be an m × n matrix. Once you specify them, you specify a single solution to the equation. So subsitute $x_2 = s,x_4 = t$ and arrive at the parametrized form:.

This is called a parametric equation or a parametric vector form of the solution. The parameteric form is much more explicit: It gives a concrete recipe for producing all solutions. Describe all solutions of $ax=0$ in parametric vector form, where $a$ is row equivalent to the given matrix. So subsitute $x_2 = s,x_4 = t$ and arrive at the parametrized form:. Parametric vector form (homogeneous case) let a be an m × n matrix. A common parametric vector form uses the free variables. You can choose any value for the free variables. As they have done before, matrix operations. Once you specify them, you specify a single solution to the equation.

This is called a parametric equation or a parametric vector form of the solution. Suppose that the free variables in the homogeneous equation ax. As they have done before, matrix operations. Parametric vector form (homogeneous case) let a be an m × n matrix. Once you specify them, you specify a single solution to the equation. The parameteric form is much more explicit: Describe all solutions of $ax=0$ in parametric vector form, where $a$ is row equivalent to the given matrix. A common parametric vector form uses the free variables. It gives a concrete recipe for producing all solutions. You can choose any value for the free variables.

Parametric form solution of augmented matrix in reduced row echelon

Suppose that the free variables in the homogeneous equation ax. Once you specify them, you specify a single solution to the equation. A common parametric vector form uses the free variables. This is called a parametric equation or a parametric vector form of the solution. So subsitute $x_2 = s,x_4 = t$ and arrive at the parametrized form:.

Example Parametric Vector Form of Solution YouTube

Suppose that the free variables in the homogeneous equation ax. As they have done before, matrix operations. So subsitute $x_2 = s,x_4 = t$ and arrive at the parametrized form:. You can choose any value for the free variables. This is called a parametric equation or a parametric vector form of the solution.

1.5 Parametric Vector FormSolving Ax=b in Parametric Vector Form

This is called a parametric equation or a parametric vector form of the solution. It gives a concrete recipe for producing all solutions. Once you specify them, you specify a single solution to the equation. So subsitute $x_2 = s,x_4 = t$ and arrive at the parametrized form:. Suppose that the free variables in the homogeneous equation ax.

Parametric Vector Form and Free Variables [Passing Linear Algebra

Suppose that the free variables in the homogeneous equation ax. You can choose any value for the free variables. Parametric vector form (homogeneous case) let a be an m × n matrix. Describe all solutions of $ax=0$ in parametric vector form, where $a$ is row equivalent to the given matrix. As they have done before, matrix operations.

202.3d Parametric Vector Form YouTube

You can choose any value for the free variables. Describe all solutions of $ax=0$ in parametric vector form, where $a$ is row equivalent to the given matrix. Suppose that the free variables in the homogeneous equation ax. So subsitute $x_2 = s,x_4 = t$ and arrive at the parametrized form:. This is called a parametric equation or a parametric vector.

Parametric vector form of solutions to a system of equations example

A common parametric vector form uses the free variables. As they have done before, matrix operations. Parametric vector form (homogeneous case) let a be an m × n matrix. Once you specify them, you specify a single solution to the equation. You can choose any value for the free variables.

[Math] Parametric vector form for homogeneous equation Ax = 0 Math

A common parametric vector form uses the free variables. It gives a concrete recipe for producing all solutions. You can choose any value for the free variables. As they have done before, matrix operations. Parametric vector form (homogeneous case) let a be an m × n matrix.

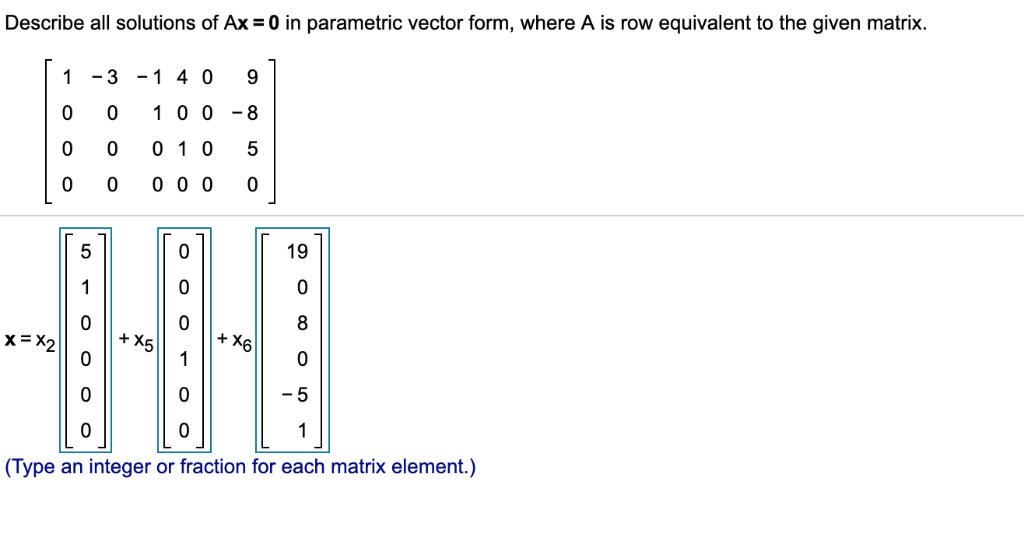
Solved Describe all solutions of Ax=0 in parametric vector

The parameteric form is much more explicit: Once you specify them, you specify a single solution to the equation. Describe all solutions of $ax=0$ in parametric vector form, where $a$ is row equivalent to the given matrix. Parametric vector form (homogeneous case) let a be an m × n matrix. As they have done before, matrix operations.

Sec 1.5 Rec parametric vector form YouTube

Suppose that the free variables in the homogeneous equation ax. The parameteric form is much more explicit: Parametric vector form (homogeneous case) let a be an m × n matrix. You can choose any value for the free variables. This is called a parametric equation or a parametric vector form of the solution.

[Math] Parametric vector form for homogeneous equation Ax = 0 Math

Suppose that the free variables in the homogeneous equation ax. So subsitute $x_2 = s,x_4 = t$ and arrive at the parametrized form:. Describe all solutions of $ax=0$ in parametric vector form, where $a$ is row equivalent to the given matrix. Parametric vector form (homogeneous case) let a be an m × n matrix. Once you specify them, you specify.

This Is Called A Parametric Equation Or A Parametric Vector Form Of The Solution.

It gives a concrete recipe for producing all solutions. So subsitute $x_2 = s,x_4 = t$ and arrive at the parametrized form:. Suppose that the free variables in the homogeneous equation ax. Describe all solutions of $ax=0$ in parametric vector form, where $a$ is row equivalent to the given matrix.

The Parameteric Form Is Much More Explicit:

Once you specify them, you specify a single solution to the equation. You can choose any value for the free variables. Parametric vector form (homogeneous case) let a be an m × n matrix. As they have done before, matrix operations.