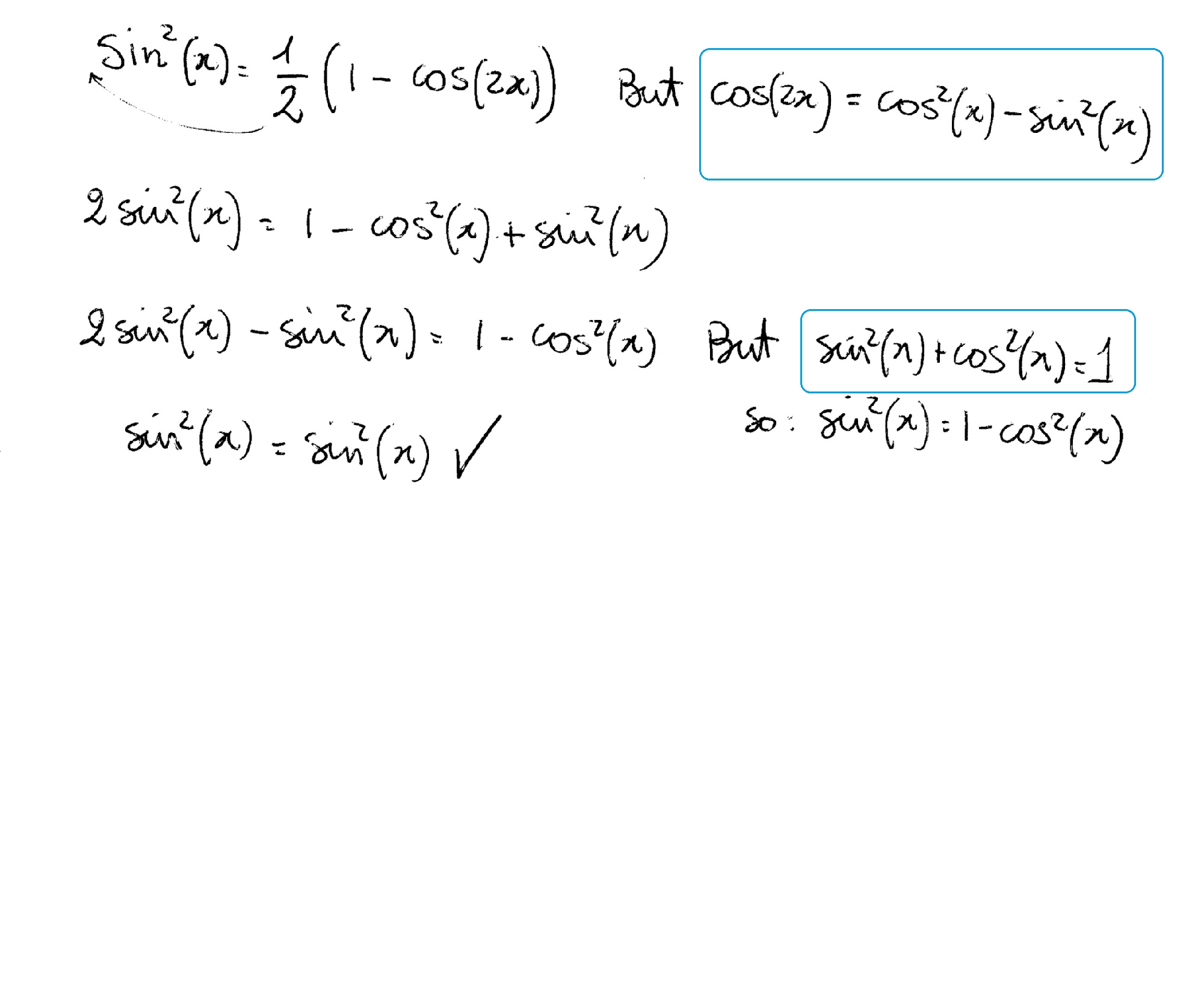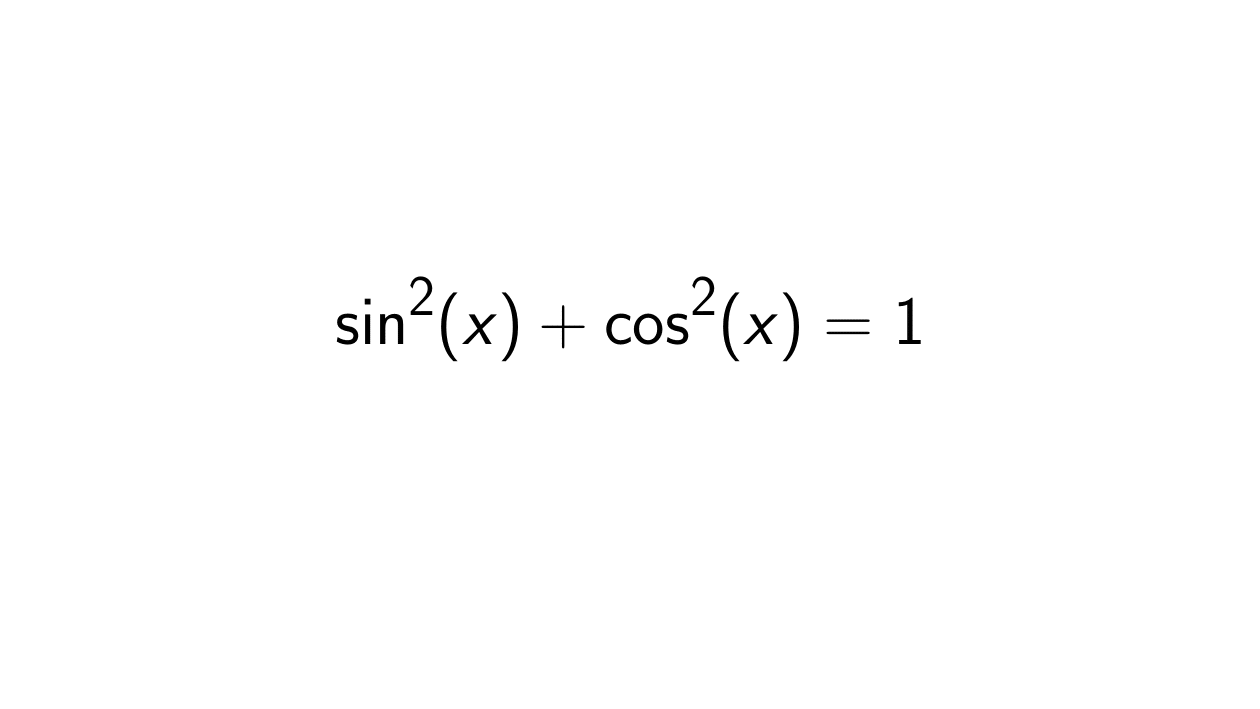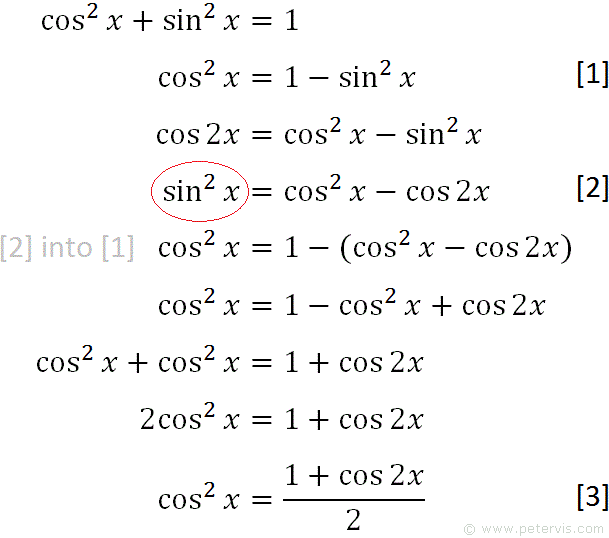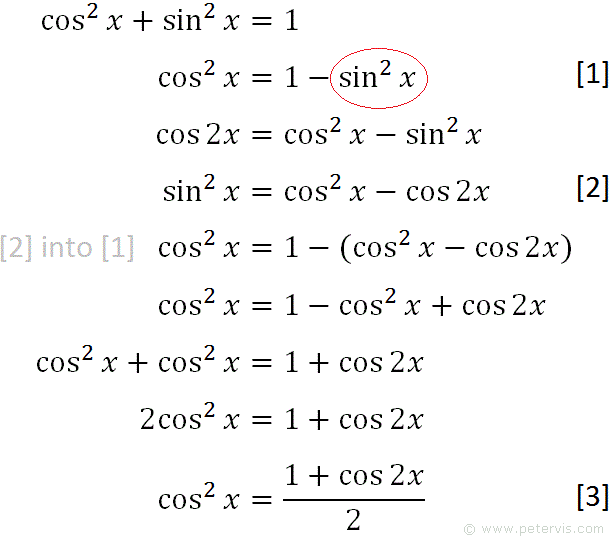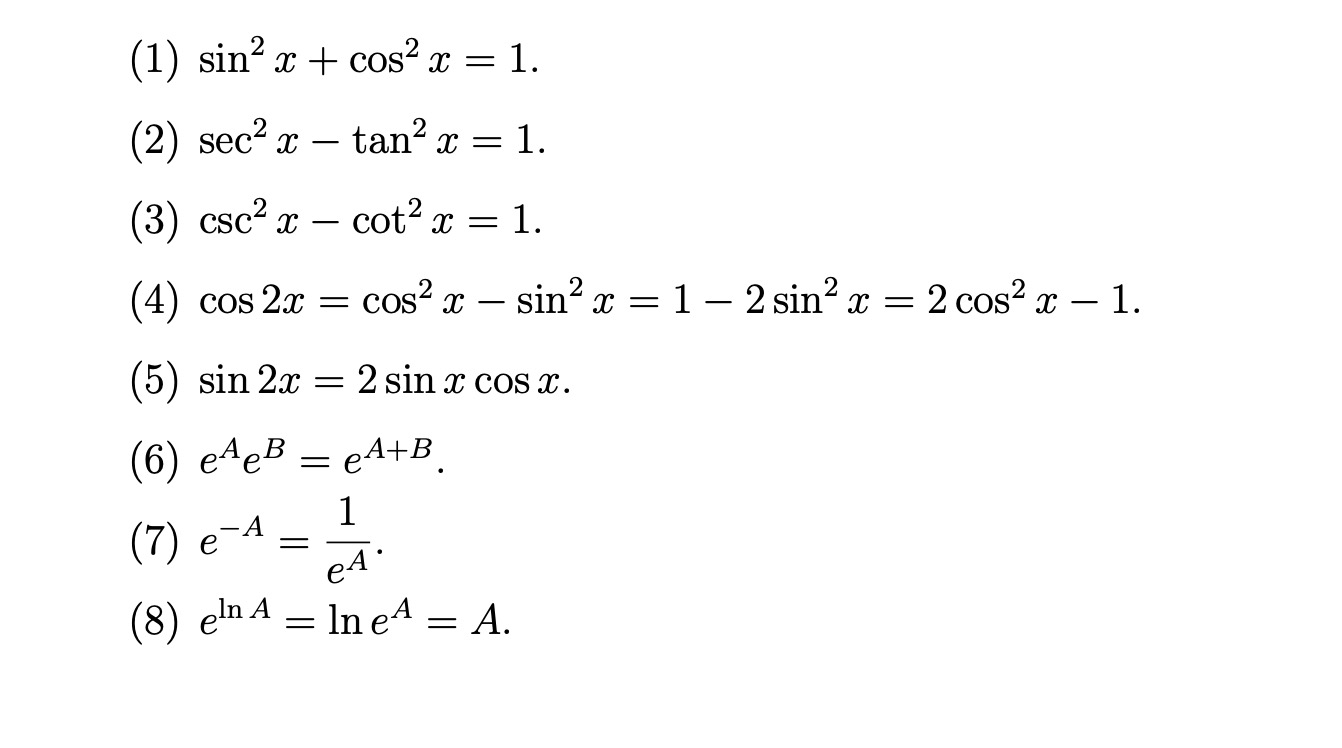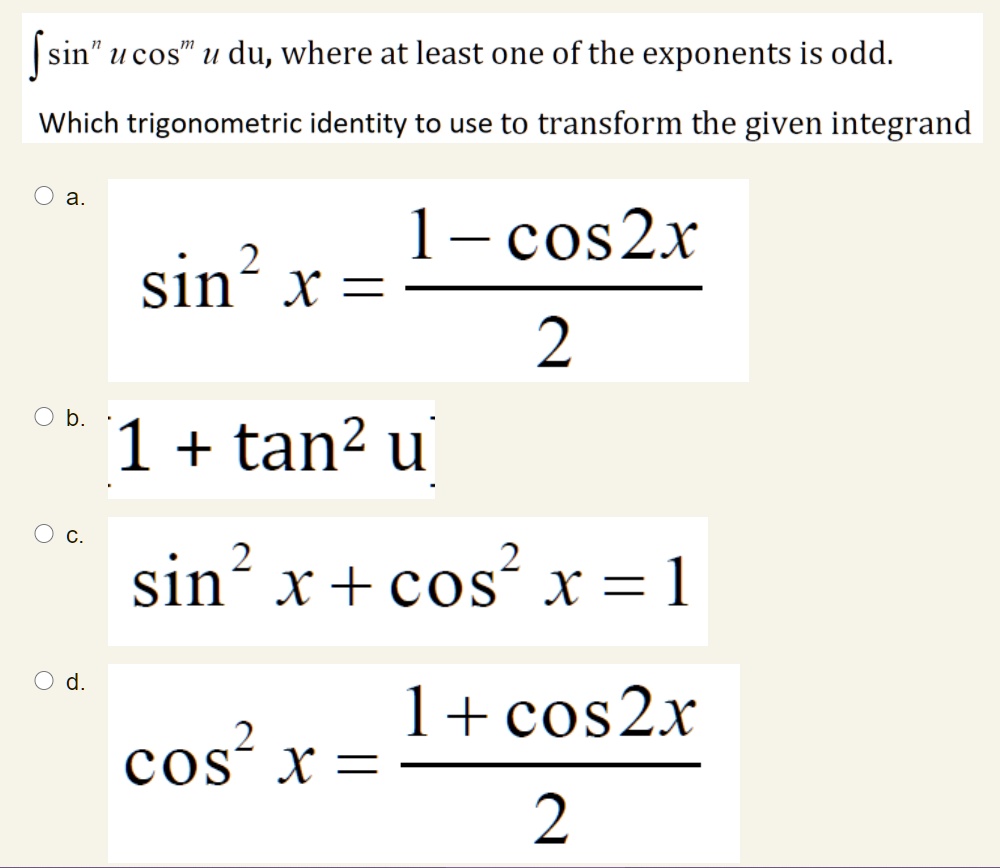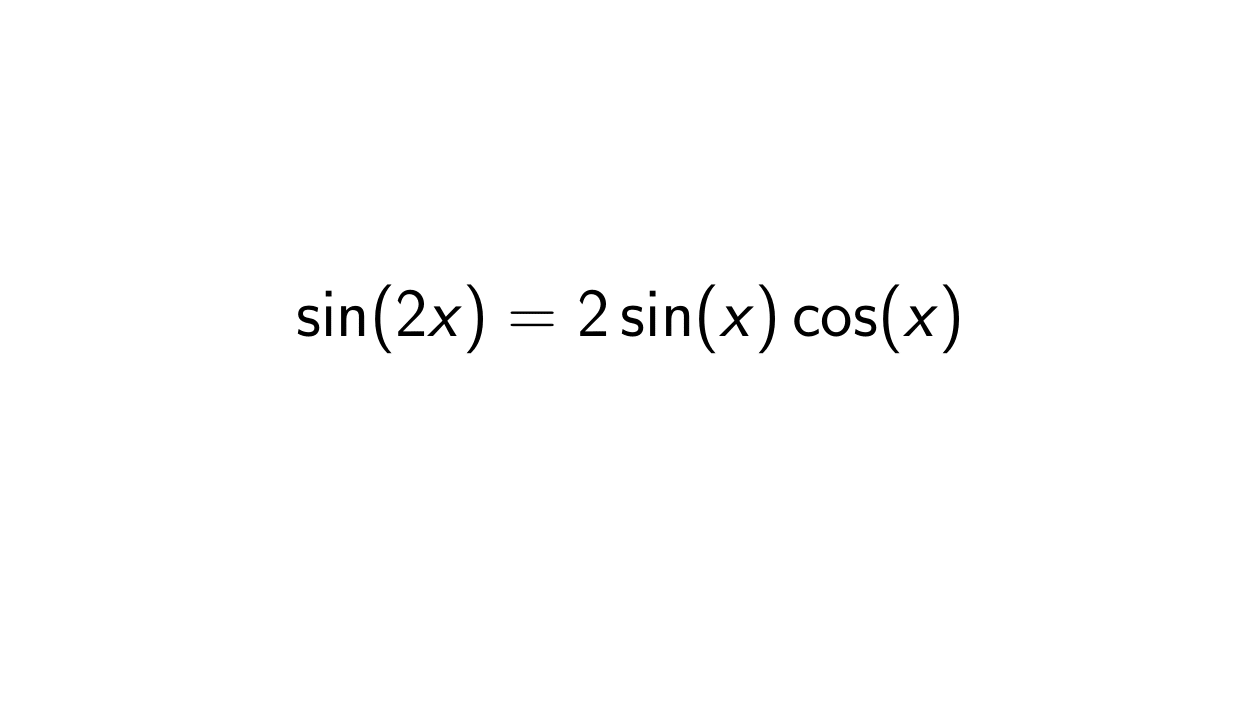Sin 2 Cos 2 1
Sin 2 Cos 2 1 - The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) = 1', a basis of many other proofs. It so happens that #sin^2(x) + cos^2(x) = 1# is one of the easier identities to prove using other methods, and so is generally. Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: Sin2x +cos2x = 1 ⇒. Just as the distance between the origin and any point (x,y) on a circle must be the circle's radius, the sum of the squared values.
Just as the distance between the origin and any point (x,y) on a circle must be the circle's radius, the sum of the squared values. Sin2x +cos2x = 1 ⇒. It so happens that #sin^2(x) + cos^2(x) = 1# is one of the easier identities to prove using other methods, and so is generally. Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) = 1', a basis of many other proofs.
Just as the distance between the origin and any point (x,y) on a circle must be the circle's radius, the sum of the squared values. Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: It so happens that #sin^2(x) + cos^2(x) = 1# is one of the easier identities to prove using other methods, and so is generally. Sin2x +cos2x = 1 ⇒. The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) = 1', a basis of many other proofs.
How do you verify sin^2(x) = (1/2)(1cos2x)? Socratic
The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) = 1', a basis of many other proofs. Just as the distance between the origin and any point (x,y) on a circle must be the circle's radius, the sum of the squared values. Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of.
Prove that sin^2(x) + cos^2(x) = 1 Epsilonify
Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) = 1', a basis of many other proofs. Sin2x +cos2x = 1 ⇒. Just as the distance between the origin and any point (x,y) on a circle must be the.
Cos 2 Sin 2 Identity on Sale
It so happens that #sin^2(x) + cos^2(x) = 1# is one of the easier identities to prove using other methods, and so is generally. Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: Just as the distance between the origin and any point (x,y) on a circle must be.
Here is a list of identities involving trigonometric
Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: Sin2x +cos2x = 1 ⇒. Just as the distance between the origin and any point (x,y) on a circle must be the circle's radius, the sum of the squared values. It so happens that #sin^2(x) + cos^2(x) = 1# is.
Solved Explain what's wrong with this proof since cos^2
Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: Sin2x +cos2x = 1 ⇒. It so happens that #sin^2(x) + cos^2(x) = 1# is one of the easier identities to prove using other methods, and so is generally. Just as the distance between the origin and any point (x,y).
Sine Formula Triangle
Just as the distance between the origin and any point (x,y) on a circle must be the circle's radius, the sum of the squared values. Sin2x +cos2x = 1 ⇒. The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) = 1', a basis of many other proofs. Replace in the equation cos2x by (1 − sin2x) we know this.
SOLVED Sin U Cos U Du, Where At Least One Of The Exponents, 48 OFF
Sin2x +cos2x = 1 ⇒. The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) = 1', a basis of many other proofs. Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: It so happens that #sin^2(x) + cos^2(x) = 1# is one of the easier identities to prove.
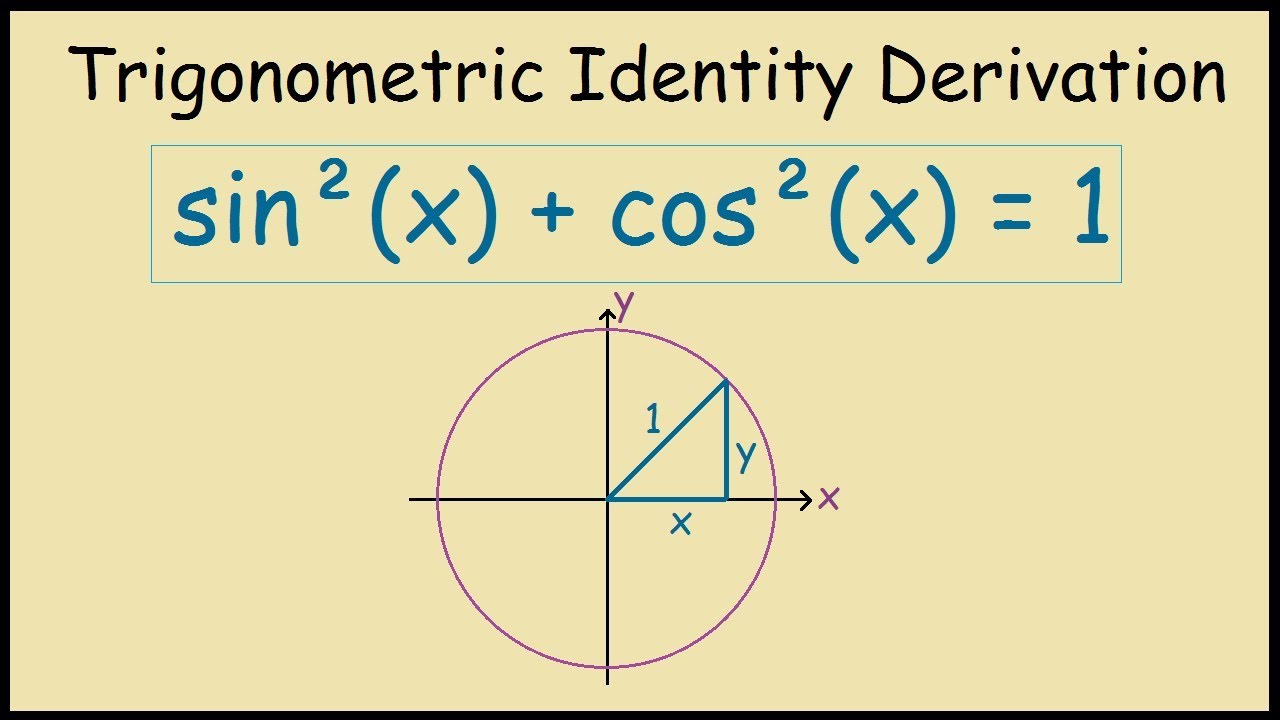
sin^2(x) + cos^2(x) = 1 Trig Identity Graphical Proof YouTube
Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: Sin2x +cos2x = 1 ⇒. It so happens that #sin^2(x) + cos^2(x) = 1# is one of the easier identities to prove using other methods, and so is generally. The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) =.
Prove that sin(2x) = 2sin(x)cos(x) Epsilonify
Just as the distance between the origin and any point (x,y) on a circle must be the circle's radius, the sum of the squared values. The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) = 1', a basis of many other proofs. It so happens that #sin^2(x) + cos^2(x) = 1# is one of the easier identities to prove.
Ex 5.6, 5 Find dy/dx, x = cos cos 2, y = sin sin 2
It so happens that #sin^2(x) + cos^2(x) = 1# is one of the easier identities to prove using other methods, and so is generally. Just as the distance between the origin and any point (x,y) on a circle must be the circle's radius, the sum of the squared values. Sin2x +cos2x = 1 ⇒. Replace in the equation cos2x by.
It So Happens That #Sin^2(X) + Cos^2(X) = 1# Is One Of The Easier Identities To Prove Using Other Methods, And So Is Generally.
Just as the distance between the origin and any point (x,y) on a circle must be the circle's radius, the sum of the squared values. The most fundamental of all trigonometric identities 'sin^2(x) + cos^2(x) = 1', a basis of many other proofs. Replace in the equation cos2x by (1 − sin2x) we know this is true through manipulation of the pythagorean identity: Sin2x +cos2x = 1 ⇒.